Description:
Explore the intricacies of rigid body dynamics in this comprehensive lecture on Euler's equations. Delve into the Newton-Euler approach, deriving the most common forms of Euler's equations for rotational dynamics of rigid bodies. Gain intuition through qualitative analysis of various examples, including spinning tops, bicycle wheels, fidget spinners, and landing gear retraction. Learn how to derive Euler's equations in body-fixed and principal axis frames, and understand their application to free rigid body motion. Conclude with simulations that bring these concepts to life, enhancing your understanding of space vehicle dynamics and rigid body behavior.
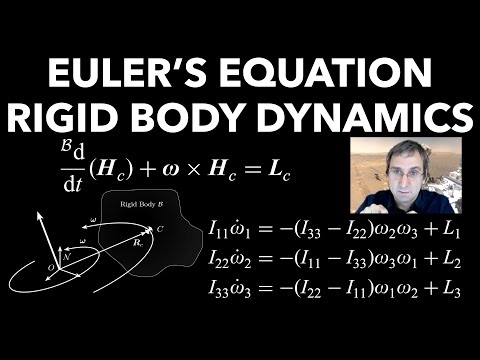
Euler's Equations of Rigid Body Dynamics Derived - Qualitative Analysis - Build Rigid Body Intuition
Add to list
#Engineering
#Aerospace Engineering
#Science
#Physics
#Mechanics
#Classical Mechanics
#Rigid Body Dynamics
#Social Sciences
#Research Methods
#Qualitative Analysis
#Spacecraft Dynamics