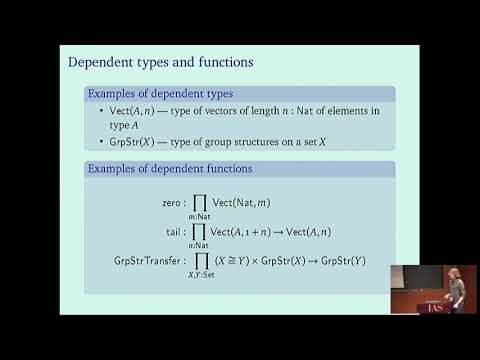
Explore univalent foundations and the equivalence principle in mathematics through this lecture from the Vladimir Voevodsky Memorial Conference. Delve into the concept of foundations in mathematics, indiscernability of identicals, and Voevodsky's homotopy lambda calculus. Examine type theory, including dependent types and functions, syntax, and inference rules. Investigate the identity type, contractible types, propositions, and sets. Learn about equivalences, path types, and axioms characterizing path types. Discover how the equivalence principle applies to set-level structures and categories. Gain insights into monoids in type theory and monoid isomorphisms. Presented by Benedikt Ahrens from the University of Birmingham, this comprehensive talk offers a deep dive into advanced mathematical concepts and their applications.