Description:
Explore the classification of Platonic solids in this mathematics lecture from the Universal Hyperbolic Geometry series. Delve into Euclid's argument from Book XIII of the Elements, using modern notations like turn angles and Schlafli symbols to examine regular polyhedra. Investigate the planar and spherical cases, discovering the five possibilities for Platonic solids. Learn about the mathematical constructions of these solids, with special attention to the tetrahedron, cube, and octahedron. Gain insights into the subtleties and challenges of Euclid's proof, and understand why the complete argument is more complex than often presented.
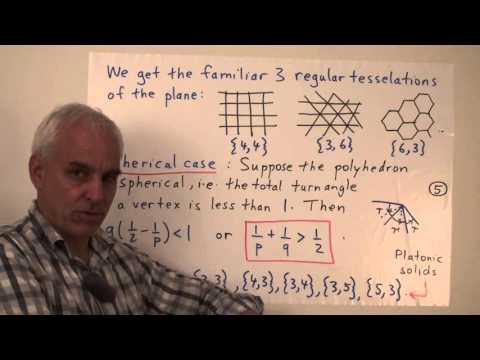
The Classification of Platonic Solids I - Universal Hyperbolic Geometry
Add to list
#Mathematics
#Geometry
#Platonic Solids
#Tessellations
#Non-Euclidean Geometry
#Hyperbolic Geometry
#Universal Hyperbolic Geometry