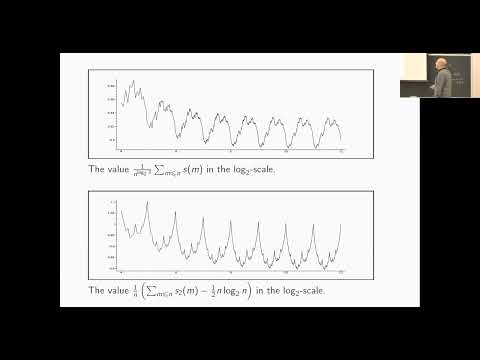
Explore the spectral theory of regular sequences in this 55-minute conference talk by Michael Coons from the University of Bielefeld. Delve into k-regular sequences, Stern's sequence, and the complexity of integer sequences. Examine the finiteness conjecture for maximal values and investigate the Zaremba sequence. Learn about ghost measures and their relation to dilation equations and iterated function systems. Discover the IFS and ghost distribution of Zaremba's sequence, and understand how to determine spectral types. Investigate continuous ghost measures and their level-set construction, with examples from the Stern sequence and 2-Zaremba sequence. Conclude by considering open questions and potential areas for further research in this field of mathematics.