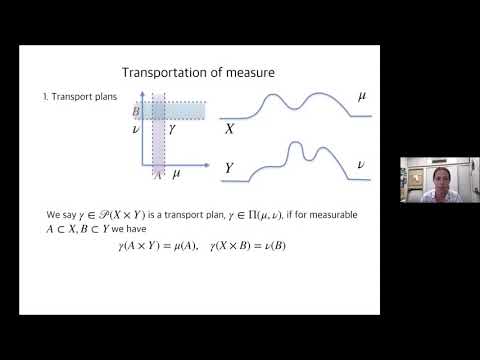
Explore optimal transport with respect to non-traditional costs in this 59-minute lecture by Shiri Artstein-Avidan from the University of Tel Aviv. Begin with a brief overview of optimal transport, introducing key concepts such as c-transform and c-subgradients. Delve into the complexities of transportation with costs that can reach infinite values, examining necessary and sufficient conditions for the existence of transport plans supported on c-subgradients (Brenier-type maps) between pairs of measures. Focus on the polar cost underlying the polarity transform for functions as a primary example. Cover topics including transport plans, quadratic cost, cyclic monotonicity, C-path boundedness, strongly C-compatible measures, Hall's Marriage Theorem, and dualities for sets. Gain insights from this joint work with Shay Sadovsky and Kasia Wyczesany, presented at the Hausdorff Center for Mathematics.