Description:
Explore nonlinear spectral decompositions in imaging and inverse problems in this SIAM-IS Virtual Seminar Series talk. Delve into a variational theory that generalizes classical spectral decompositions in linear filters and singular value decomposition of linear inverse problems to a nonlinear regularization setting in Banach spaces. Examine applications in imaging and data science, and learn about computing nonlinear eigenfunctions using gradient flows and power iterations. Cover topics such as basic inverse problems, optimization, constraint qualification, variation analysis tools, normal cones, optimality systems, directional differentiability, boolean subdifferential theorem, nonlocal models, functional analysis, kernel estimation, and implementation details. Gain insights from speaker Martin Burger of FAU as he presents "Nonlinear spectral decompositions in imaging and inverse problems" in this 59-minute seminar.
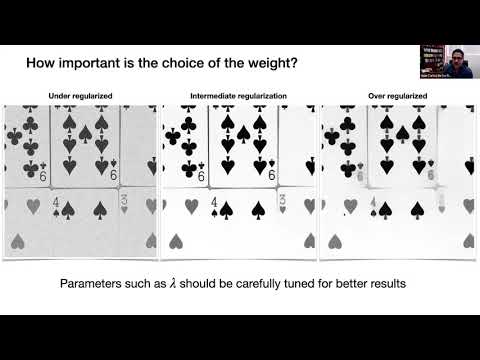
Nonlinear Spectral Decompositions in Imaging and Inverse Problems
Add to list