Description:
Explore the application of discrete Morse theory in computing the rank invariant for multi-parameter persistence modules in this 54-minute lecture. Delve into how critical points, determined by a discrete Morse function, partition the parameter space into equivalence classes and dictate the behavior of the rank invariant. Learn to deduce persistence diagrams for entire classes of rank invariants from a single representative, and understand the importance of critical values in multi-parameter filtrations. Gain insights into the computation of rank invariants through fibration and the creation of equivalence classes of lines, ultimately establishing bijections between diagrams along equivalent lines.
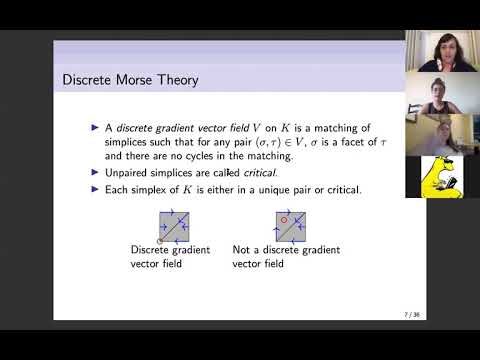
Morse-Based Fibering of the Rank Invariant
Add to list
#Mathematics
#Geometry
#Topology
#Algebraic Topology
#Data Science
#Data Analysis
#Calculus
#Critical Points
#Topological Data Analysis
#Persistence Diagrams
#Discrete Mathematics
#Discrete Morse Theory