Description:
Explore the fascinating world of Algebraic Calculus and de Casteljau Bezier (dCB) curves in this 54-minute lecture. Delve into a new approach to calculus that focuses on polynomially parametrized curves, essential in design, animation, architecture, and industrial applications. Learn about polynumbers, R points, and the quadratic and cubic cases of dCB curves. Discover new formulas for planar integration and engage with research-level mathematics using GeoGebra to demonstrate de Casteljau's algorithm. Examine the linear algebra of Pascal matrices and their inverses, and understand the dCB parametrization theorem. Gain insights into this exciting field that connects abstract mathematical concepts with computational reality through concrete examples and calculations.
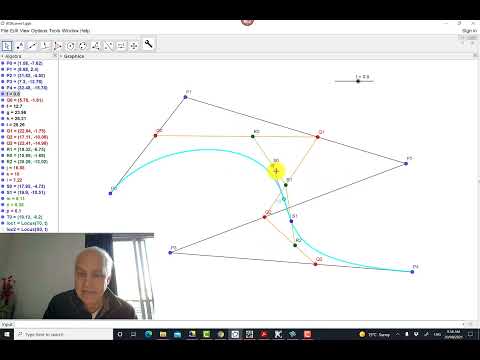
Polynumbers and De Casteljau Bezier Curves - Algebraic Calculus and DCB Curves - N J Wildberger
Add to list