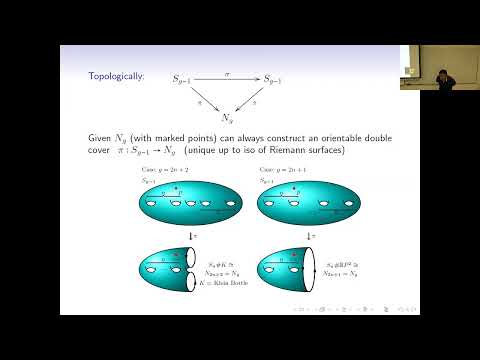
Explore a comprehensive lecture on the Nielsen realization problem and its implications for non-orientable surfaces with marked points. Delve into the proof that Nielsen realization holds for these surfaces, contrasting it with the lack of a section from the group of diffeomorphisms to the mapping class group for large genus. Examine the concept of fixed point data for diffeomorphisms of non-orientable surfaces and its applications in classifying normalizers of cyclic subgroups and studying p-periodicity. Discover how these findings contribute to determining the p-primary component of Farrell cohomology for mapping class groups in specific cases. Gain insights into advanced topics such as Kirchhoff's theorem, client surfaces, community diagrams, and the analog of the Attack Miller space throughout this in-depth mathematical exploration.