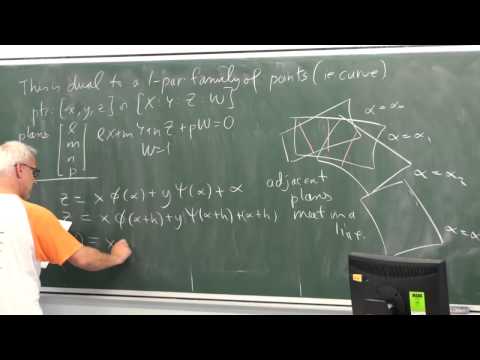
Explore the contributions of Gaspard Monge, a pioneering French differential geometer, in this 48-minute lecture. Delve into Monge's inventions, including descriptive geometry and its military applications. Examine his theorems in Euclidean geometry, such as homothetic centers of three circles and the Monge point of a tetrahedron. Investigate Monge's work on curves, families of surfaces, edges of regression, and lines of curvature. Learn about developable surfaces, one-parameter families of surfaces, and lines of curvature on circles and ellipsoids. Gain insights into the trigonometry of tetrahedra and review key concepts in plane geometry to enhance your understanding of differential geometry.