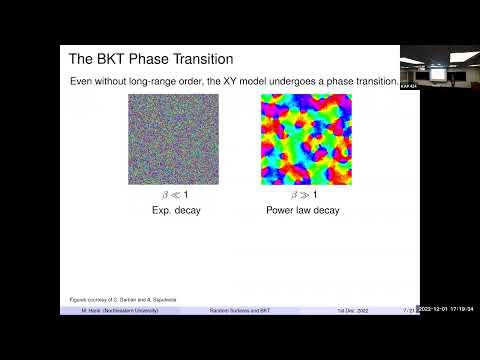
Explore a 57-minute lecture from the USC Probability and Statistics Seminar featuring Matan Harel discussing the delocalization of planar integer-valued height functions. Delve into the relationship between two-dimensional lattice models, focusing on O(2)-invariant spin models and integer-valued height function models. Learn how delocalization in height function models implies power-law decay in associated O(2)-invariant spin models. Discover the extension of Lammers' work showing delocalization of certain integer-valued height functions on doubly periodic graphs. Gain new insights into the Berezinksii-Kosterlitz-Thouless phase transition for two-dimensional O(2)-invariant lattice models. Follow the comprehensive syllabus covering topics such as the Ising Model, XY Model, continuous symmetry, Mermin-Wagner theorem, Peierls vs. Spin Wave, integer-valued random surfaces, localization and delocalization, duality relations, lattice monotonicity, and the connection between level lines and slow decay. Read more