Description:
Explore a groundbreaking framework for constructing coordinates in finite Lens spaces for data with nontrivial 1-dimensional persistent cohomology in this 27-minute conference talk. Delve into the innovative approach of defining coordinates on an open neighborhood of the data using only a small subset of landmarks. Discover the newly introduced dimensionality reduction scheme, LPCA, and witness its effectiveness when combined with persistent cohomology and Lens coordinates for nonlinear topological dimensionality reduction. Examine the stability of Lens coordinates under Wasserstein perturbations to the landmark set, and gain insights into the bounds for coordinate changes in relation to perturbation and landmark distribution.
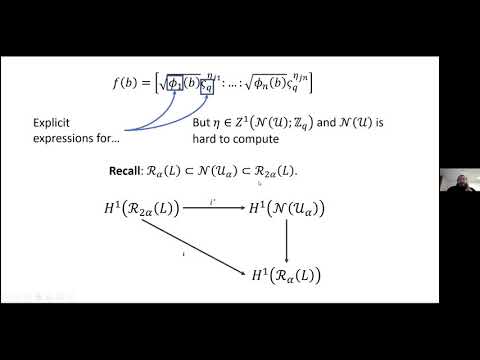
Data Driven Torsion Coordinates and Wasserstein Stability
Add to list
#Mathematics
#Geometry
#Topology
#Data Science
#Data Analysis
#Computer Science
#Machine Learning
#Dimensionality Reduction