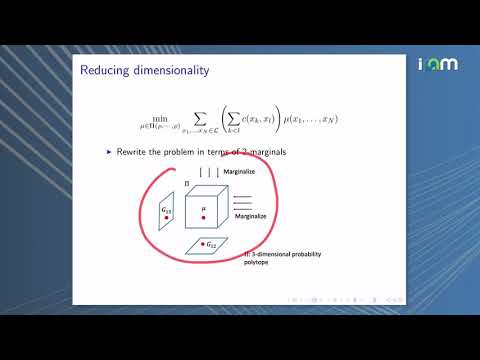
Explore a 29-minute lecture on "Strictly-correlated Electron Functional and Multimarginal Optimal Transport" presented by Lexing Ying from Stanford University at the Tensor Methods and Emerging Applications to the Physical and Data Sciences 2021 workshop. Delve into the application of convex optimization methods for solving multi-marginal transport problems in density functional theory. Learn about convex relaxations providing outer approximations to N-representable 2-marginals and 3-marginals, resulting in lower energy bounds. Discover proposed rounding schemes for obtaining upper energy bounds. Follow the lecture's structure, covering topics such as the Schrodinger equation, problem motivation, canonical basis, objective function, concave relaxation, and numerical examples. Gain insights into this advanced mathematical approach presented at the Institute for Pure and Applied Mathematics, UCLA.