Description:
Explore the fascinating world of algebraic geometry in this 46-minute lecture by Jacob Lurie at the Hausdorff Center for Mathematics. Delve into the Riemann-Hilbert correspondence and its implications for p-adic geometry. Trace the historical development from Hilbert's 21st problem to the groundbreaking work of Kashiwara and Mebkhout. Examine the challenges of translating this correspondence to non-archimedean fields like Qp. Discover recent advancements in prismatic cohomology and their potential applications. Cover key concepts including Fuchsian systems, monodromy representations, local systems on complex manifolds, algebraic D-modules, and the de Rham complex. Gain insights into the intersection of topology, algebraic differential equations, and complex algebraic varieties in this comprehensive exploration of modern mathematical theory.
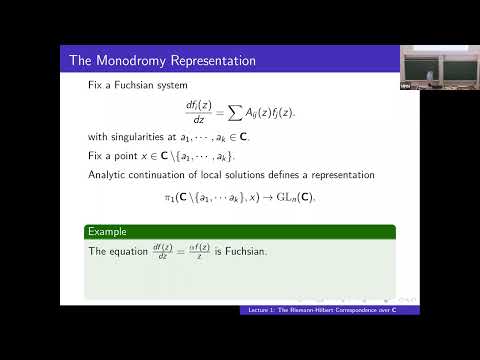
Jacob Lurie: A Riemann-Hilbert Correspondence in P-adic Geometry Part 1
Add to list
#Mathematics
#Algebraic Geometry
#Differential Equations
#Complex Analysis
#Monodromy
#Prismatic Cohomology
#Number Theory
#p-adic Geometry