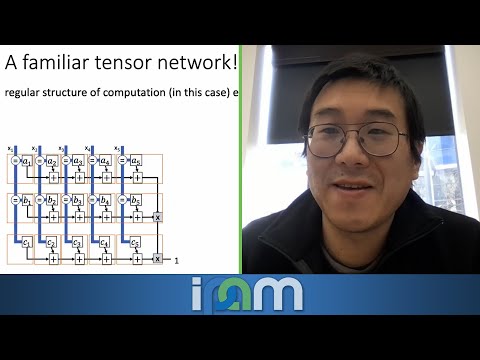
Explore a 32-minute lecture on arithmetic tensor networks and integration presented by Garnet Chan from the California Institute of Technology at IPAM's Quantum Numerical Linear Algebra Workshop. Delve into the intricacies of performing arithmetic with tensor networks and its implications for function integration. Examine topics such as tensor network contraction, approximate contraction techniques, and their applications in multivariable function integration. Discover how arithmetic circuit representations and tensor network circuits can be utilized for polynomial integration, and investigate the relationship between exact and approximate contractions. Gain insights into the dependence of integration on various factors, including the number of variables and the nature of the integrand. Conclude with a discussion on Gaussian integration in a hypercube, providing a comprehensive overview of this advanced mathematical concept.