Description:
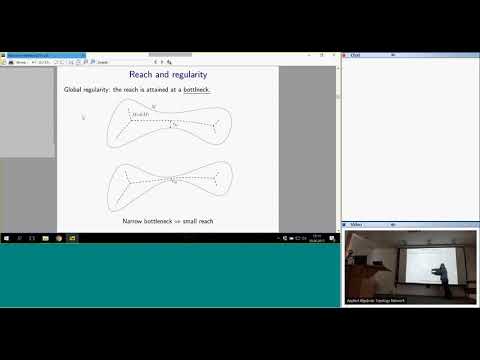
Explore the intricacies of manifold estimation and geometric inference in this lecture on estimating the reach of a manifold. Delve into the concept of reach, also known as conditioning number or feature size, and its role in measuring manifold regularity. Learn about a proposed estimator for reach when tangent spaces are known, and examine upper and lower bounds on minimax rates for reach estimation. Investigate problem statements, geometric and statistical models, loss functions, and the third order condition. Analyze minimax estimates in global and local cases, and consider Le Cam's Lemma heuristic. Address challenges when tangent spaces are unknown and explore tangent space stability. Gain insights into ongoing research and future directions in this field of applied algebraic topology.
Estimating the Reach of a Manifold
Add to list