Главная
Study mode:
on
1
Understanding the Definition of the Derivative
2
Sketching the Derivative of a Function
3
Finding a Derivative Using the Definition of a Derivative
4
Derivative Using the Definition, Example 2
5
Instantaneous Velocity Using Limit Definition of Derivative
6
Instantaneous Velocity, Definition of Derivative
7
Limit Definition of Derivative, Rational Function Example
8
Derivative Using Definition: Rational Function Example
9
Limit Definition of Derivative, Square Root Example
10
Derivative of Root Function Using Definition of a Derivative
11
Basic Derivative Rules - The Shortcut Using the Power Rule
12
Power Rule and Derivatives, A Basic Example #1
13
Power Rule and Derivatives, A Basic Example, #2
14
Power Rule for Finding Derivatives, Basic Example #3
15
Basic Derivative Examples
16
More Derivative Examples, #1
17
More Derivative Examples, #2
18
More Derivative Examples, #3
19
The Product Rule for Derivatives
20
Derivatives using the Product Rule
21
Proof of the Product Rule from Calculus
22
The Quotient Rule
23
Derivatives Using the Quotient Rule
24
General Chain Rule , Partial Derivatives - Part 1
25
General Chain Rule - Part 2
26
Finding Derivatives using the Chain Rule
27
Chain Rule for Finding Derivatives
28
Basic Chain Rule Problems
29
More Chain Rule Examples #1
30
More Chain Rule Examples #2
31
More Chain Rule Examples #3
32
Using the Chain Rule - Harder Example #1
33
Using the Chain Rule - Harder Example #2
34
Using the Chain Rule - Harder Example #3
35
Derivatives - Product + Chain Rule + Factoring
36
Product Rule, Chain Rule and Factoring - Ex 2
37
Derivatives - Quotient and Chain Rule and Simplifying
38
More Complicated Derivative Examples
39
More Complicated Derivative Examples
40
❖ Lots of Different Derivative Examples! ❖
41
Implicit Differentiation - Basic Idea and Examples
42
Implicit Differentiation - Basic Example 1 / 3
43
Implicit Differentiation - Basic Example 2 / 3
44
Implicit Differentiation - Basic Example 3 / 3
45
Using Implicit Differentiation - Extra Examples
46
Using Implicit Differentiation - Example
47
Using Implicit Differentiation to find a derivative
48
Using Implicit Differentiation to find a Second Derivative
49
Implicit Differentiation - More Examples
50
More! Implicit Differentiation Examples
51
More Implicit Differentiation Examples - 3
52
❖ Derivatives of Logarithmic Functions ❖
53
Derivatives of Logarithm Functions - 1 of 2
54
Derivatives of Logarithm Functions - 2 of 2
55
Derivatives of Logarithmic Functions and Examples
56
Derivatives of Logarithmic Functions - More Examples
57
Logarithmic Differentiation
58
Logarithmic Differentiation - Example 2
59
Logarithmic Differentiation - Example 3
60
Derivatives of Exponential Functions
61
Derivatives of Exponential Functions
62
Derivatives of Exponential Functions and Examples
63
Exponential Functions and Derivatives, More Example #1
64
Derivatives of Parametric Functions
65
Derivatives of Parametric Equations, Another Example #1
66
Derivatives of Parametric Equations, Another Example #2 - Second Derivative
67
Parametric Curves - Finding Second Derivatives
68
More Derivatives Involving Trigonometric Functions, Ex 1
69
More Derivatives Involving Trigonometric Functions, Ex 2
70
Deriving the Derivative Formulas for Tangent, Cotangent, Secant, Cosecant
71
Inverse Trigonometric Functions - Derivatives
72
Derivatives of Inverse Trigonometric Functions - Another Example!
73
Deriving the Derivative of Inverse Tangent or y = arctan (x)
74
Derivative of a Vector Function - Another Ex 1
75
Inverse Trigonometric Functions - Derivatives - Example 2
76
Inverse Trigonometric Functions - Derivatives - Example 3
77
Hyperbolic Functions - Derivatives
78
Inverse Hyperbolic Functions - Derivatives
79
Position, Velocity, Acceleration using Derivatives
Description:
Explore a comprehensive tutorial on derivatives, covering the definition, basic examples, and various rules including product, quotient, and chain rules. Delve into complex topics such as implicit differentiation, logarithmic differentiation, and derivatives of exponential, parametric, and trigonometric functions. Learn to sketch derivatives, solve instantaneous velocity problems, and apply the power rule. Master techniques for handling complicated derivative examples, including those involving factoring and simplification. Discover the applications of derivatives in position, velocity, and acceleration calculations, as well as in inverse trigonometric and hyperbolic functions.

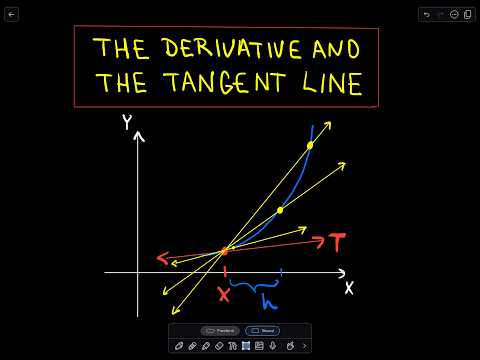
Derivatives

patrickJMT
Add to list
#Mathematics #Calculus #Business #Finance #Trading #Derivatives #Chain Rule #Trigonometric Functions #Exponential Functions #Logarithmic Functions #Implicit Differentiation #Product Rule #Quotient Rule
1 of 79

Understanding the Definition of the Derivative