Description:
Explore the algebraic structure of the Euclidean projective line in this 41-minute mathematics lecture. Delve into the fundamental projective Triple quad formula and its proof, examine the equal p-quadrances theorem, and discover how the logistic map from chaos theory emerges as the second Spread polynomial. Investigate rotations and reflections in one dimension, and observe how the composition of rotations naturally leads to an algebraic structure on the projective line, analogous to complex number multiplication. Learn about isometries, the Euclidean projective line, and the rotation isometry theorem while gaining insights into advanced geometric concepts and their applications.
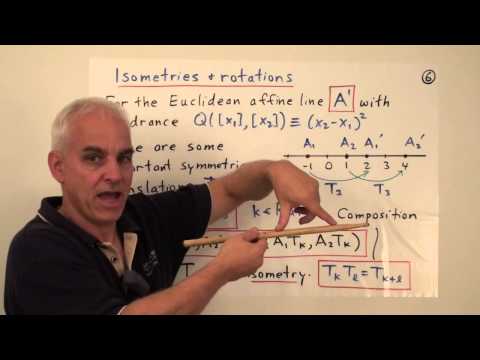
Algebraic Structure on the Euclidean Projective Line - Rational Geometry Math Foundations 137
Add to list
#Mathematics
#Geometry
#Projective Geometry
#Complex Numbers
#Chaos Theory
#Algebra
#Algebraic Structures
#Euclidean Geometry