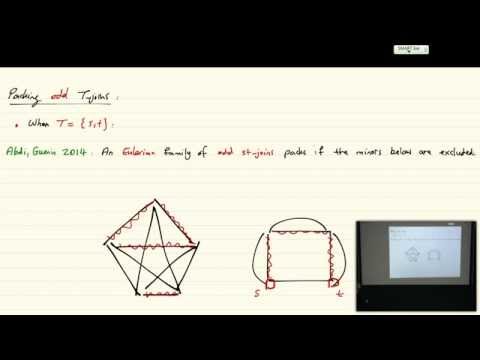
Explore a lecture on packing odd T-joins with at most two terminals, delivered by Ahmad Abdi at the Hausdorff Center for Mathematics. Delve into the intricacies of graph theory as Abdi presents a theorem developed with Guenin, which establishes conditions for equality between maximum packing size and minimum cover size of edge-disjoint odd T-joins. Discover how this result extends Guenin's characterization of weakly bipartite graphs and its applications in packing two-commodity paths, T-joins with up to 4 terminals, and covering edges using cuts. Follow Abdi's overview of the proof, which incorporates Menger's theorem, the 2-linkage theorem, and disentangling techniques. Gain insights into the lecture's structure, covering introduction, problem theme, parity conditions, applications, proof strategy, and open questions in this 32-minute presentation from the Hausdorff Trimester Program on Combinatorial Optimization.